

Developing Problem Solvers For The 21st Century
By Associate Professor Lee Ngan Hoe, Mathematics and Mathematics Education Academic Group
Using tools like the problem wheel, teachers can help students become better maths problem solvers by expressing their abstract thought processes in tangible forms.
Today’s educators will generally acknowledge that higher order thinking skills, such as critical and creative thinking skills, play an important role in the students’ success in mathematical problem-solving. However, there has not been as much emphasis on the students’ meta cognition abilities despite several researches that showed the importance of developing the students’ metacognitive skills to enhance their success in problem-solving.
Commonly referred to as ‘thinking about thinking’, metacognition is a kind of ‘superordinate thinking’. It involves heightened awareness to active monitoring, and a constant regulation of one’s thinking during problem-solving. Meta cognition has also been identified as one of the key brain activities that separates an expert problem solver from a novice one. Unsurprisingly, metacognition is often cited as one of the key 21st century competencies essential in an era where people will be facing more novel and complex situations. These situations are not unlike the problem-solving scenarios we have provided to our students in the classroom.
The abstractness of thinking makes it a challenging task to develop thinking, and even more so for superordinate thinking. One way to develop students’ metacognitive skills is by making their thinking process tangible, either visually or aurally. This allows them to engage in ‘productive thinking’ as they monitor, reflect and regulate their thought process while tackling a task on hand.
Due to in experience, many students will resort to ‘impulsive thinking’ instead. The senovice problem solvers, who tend to approach problem-solving linearly and based on first impression, will feel daunted when they are unable to find an immediate solution to the problem. However, problem-solving is often a learning journey. A task is a problem to a given solver because there exists an obstacle between the knowns and the unknowns within the task, as shown in the diagram below.
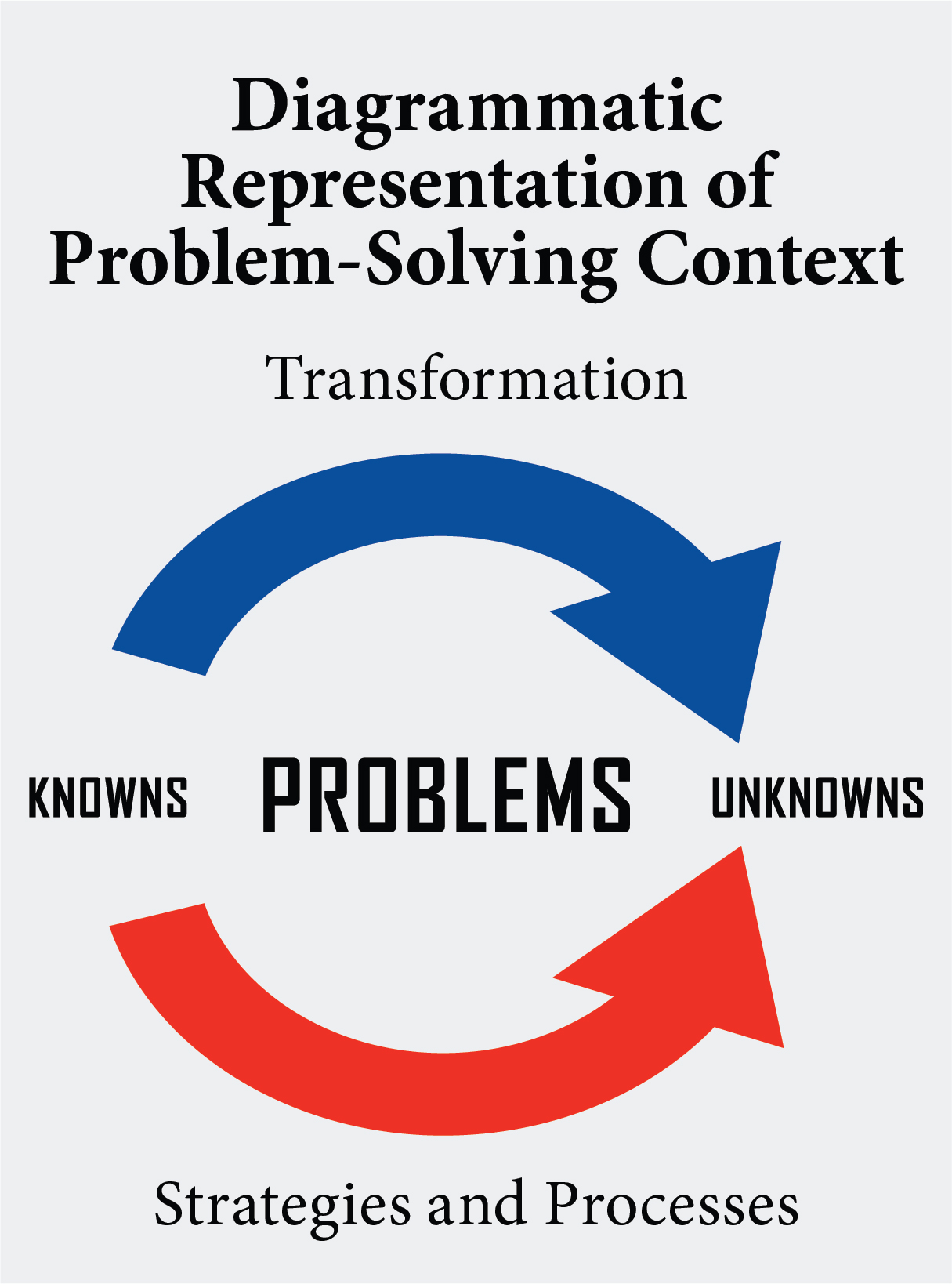
During the problem-solving journey, students often need to transform contextual information into mathematical language and ideas to facilitate their use of mathematical knowledge and to make sense of the problem on hand. They learn to employ strategies and processes to manoeuvre from the given known(s) towards the unknown(s) within the task so that they can determine a pathway to solve the problem. The solution is thus not always obvious to the solvers at first blush.
Teachers can help the students develop productive thinking and avoid impulsive thinking by cultivating metacognitive habits in the students where they can become more aware of their thinking processes, and thereby, monitor and regulate the way they reason and approach problem-solving.
Just like physical habits, habits of the mind can be nurtured through consistent practice in rich and relevant contexts. One such method is to use the Problem Wheel and the accompanying generic question prompts, shown in diagram below,to help students develop a metacognitive approach to problem-solving and focus their thinking on understanding and planning a pathway to solving the problem.

| Component of Problem Wheel | Examples of Question Prompts | Student’s Response |
|---|---|---|
| Given | • What is / are given to us in this problem? • What do we know about the problem? • What value(s) is / are we given to us in this problem? | |
| Find | • What are we supposed to find in this problem? • Which value(s) is /are we supposed to find as the answer to this problem? | |
| Picture | • Can we draw a picture to represent this problem? • What would we draw to represent this problem? | |
| Topic | • Which is / are the topic(s) we have learnt that might help us solve this problem? | |
| Formula(e) | • Which is / are the formula(e) we have learnt that would help us to solve this problem? |
Teachers can encourage the students to use the Problem Wheel in the classrooms to solve maths problems either individually or in small groups. By making their thinking processes tangible, it will help them monitor and regulate their thinking. The question prompts should eventually be removed to allow students to form the habit of asking the questions intuitively on their own. In time, the students will appreciate problem-solving as a journey where the solution is just an end-product.
The approach to encourage productive thinking in students is not only useful for teachers to develop metacognitive problem solvers in the 21st century, it is a tool that parents,as active partners to their children’s mathematical learning journey, can use at home too. Rather than providing their children with model answers and depriving them of the opportunities to develop their productive thinking habits, parents can use the Problem Wheel approach to prepare and develop their children’s metacognitive skills instead.
Loving those maths problems!
Here’s a four-part series by a local newspaper that serves as a good example of how parents and children could engage and have fun solving maths problems together.
Week 1 - http://www.straitstimes.com/singapore/education/fun-with-mathematics-question-1
Week 2 - http://www.straitstimes.com/singapore/education/fun-with-mathematics-question-2
Week 3 - http://www.straitstimes.com/singapore/education/fun-with-mathematics-question-3
Week 4 - http://www.straitstimes.com/singapore/education/fun-with-mathematics-question-4





















